Dan Johns thinks about how Archimedes' method to approximate Pi (π) is relevant to the BLOODHOUND SSC design process. Our contribution to International Pi Day.
Einstein said of splitting the atom by bombardment that it is as hard to achieve as “shooting birds in the dark in a place where there are only a few birds”. This is also true of achieving a design for 133,000hp land-vehicle being accelerated to 1000mph without the discovery of π - the ‘Design Process’ would be a bit hit or miss.
When designing something that has never been done before, there are very few reference points to work from, although having the experience from Thrust SSC arguably is a good starting point. However we are still starting with a blank sheet of paper, as Thrust SSC was not a 1000mph car and therefore we still have many unknowns and unknown variables to solve as we progress through the design of the vehicle.
A design process is a process that finds answers to problems by using experimentation, and optimisation results, to help narrow all the variables to converge on a solution (or not). The first part of the process is to define boundaries ‘around’ where the answer may lay - Create the ‘Design Space’ and make assumptions as to where ‘X’ marks the spot.
Recently I've been working on explaining 'Design Theory'. At the heart of this I am discussing experimental design techniques which help us navigate to where the answer might lay within a 'Design Space' by using statistical trend analysis. Put simply we use trial and error to navigate with multiple variables, which I often equate to being a lot like trying to resolve a 3D Killer Suduko. We set a boundary condition with quantifiable known elements and then iterate variables within that space, and keep refining the boundary as more known elements are found along the way.
We then get to a point where all 'practical possibilities' converge without looking for, or needing, a finite answer, which is something Neil Armstrong reinforced when we met him in December 2010. His experiences with scientist and engineers highlighted what industry now calls ‘on-quality’ or put another way ‘fit for purpose’. A lot of the time engineering is about 'close enough is good enough', which is a point sometimes lost in teaching undergraduates. Why work to 6 sig. figs. when 2 will do?
This approach is pretty much how Archimedes found π 2000 years ago. He used 2 known quantifiable hexagons, one just touching inside and the other just touching outside the circle. One hexagon was bigger than the other and so he could calculate the perimeter of each hexagon, and know that the answer to π lay between the two results (see diagram below). This method got him to about 90% accuracy. Then by increasing the number of sides to 96 (refining the boundary) he got to within 99.9% accuracy and therefore good enough. If he progressed to 524288 sides, then he would have reached the limits of an Excel spreadsheet which reports 100%.
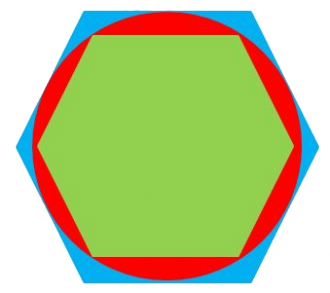
Archimedes' approximation of π
The circumference of the red circle is somewhere between that of the blue and green hexagons
This method is called the 'Squeeze Theorem' and we use it implicitly in the design tools and processes for functions such as multi-objective modelling and topology optimisations, which are both iterative processes to refine a best guess.
Archimedes' process for finding π has taught us a few things that we use today in our design philosophy:
- We start off with a best guess (what does the car need to look like? Best guess = Concept 1)
- Refine the boundary conditions and 'squeeze' the variables (best guess made better = Concept 2) and so on.
- Set a limit on the number of refinements. Getting to 99% is good enough. Don't chase 'ultimate π' as we'll spend too much time trying to get a 1% improvement when what we have offers 'all practical possibilities'.
- Close enough is good enough.
Because a finite answer is not what we are looking for, it leaves much more scope for many possible solutions and why Land Speed Record cars can all look very different.
If Microsoft had been around in 250BC, and if Archimedes had had a shot gun, I bet he still would have found a way to shoot birds in the dark without the need for a spreadsheet!
